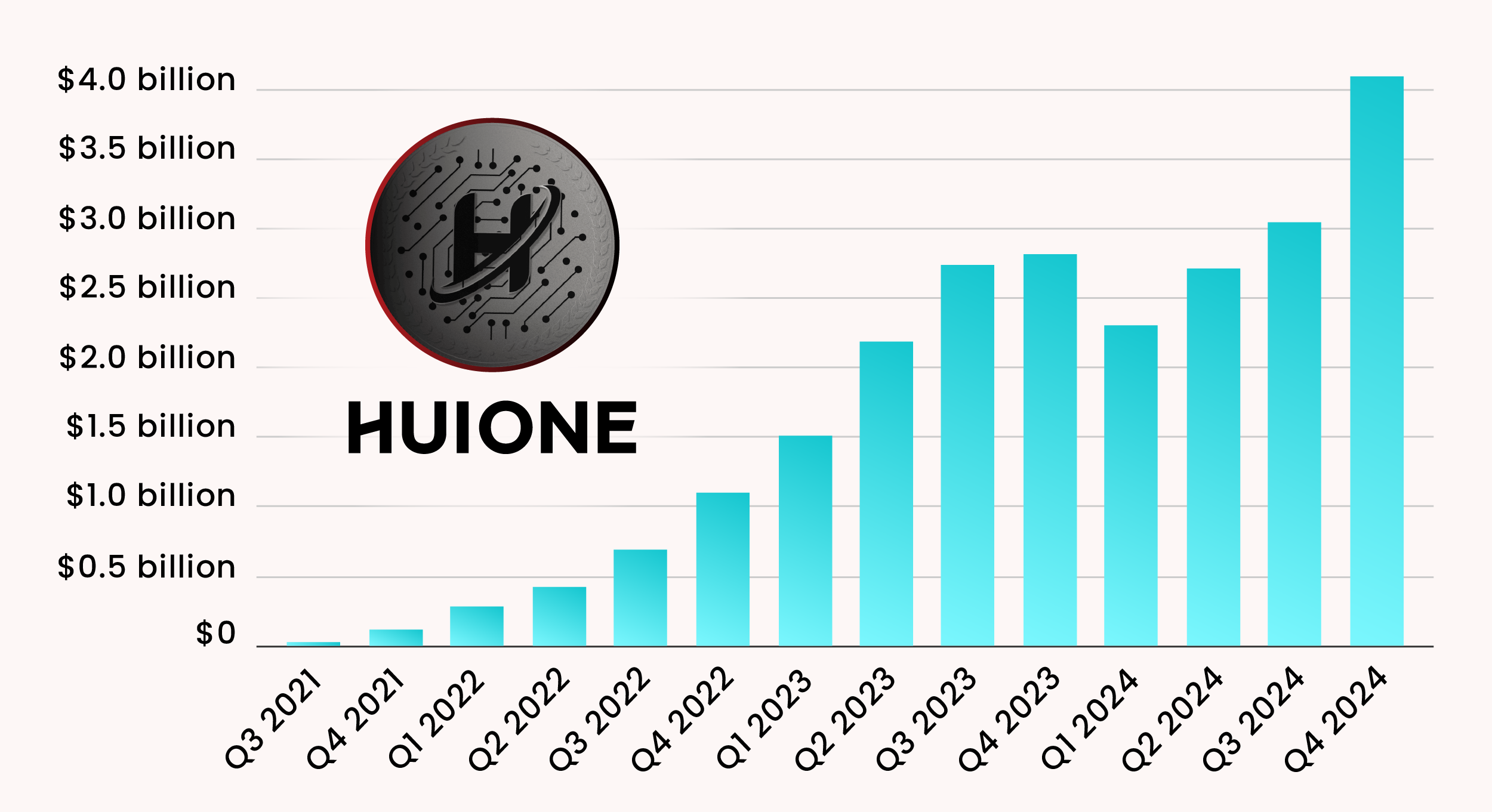
This Elegant Math Problem Helps You Find the Best Choice for Hiring, House-Hunting and Even Love
Math’s “best-choice problem” could help humans become better decision-makers, at everything from choosing the best job candidate to finding a romantic partner
Imagine cruising down the highway when you notice your fuel tank running low. Your GPS indicates 10 gas stations lie ahead on your route. Naturally, you want the cheapest option. You pass the first handful and observe their prices before approaching one with a seemingly good deal. Do you stop, not knowing how sweet the bargains could get up the road? Or do you continue exploring and risk regret for rejecting the bird in hand? You won’t double back, so you face a now-or-never choice. What strategy maximizes your chances of picking the cheapest station?
Researchers have studied this so-called best-choice problem and its many variants extensively, attracted by its real-world appeal and surprisingly elegant solution. Empirical studies suggest that humans tend to fall short of the optimal strategy, so learning the secret might just make you a better decision-maker—everywhere from the gas pump to your dating profile.
The scenario goes by several names: “the secretary problem,” where instead of ranking gas stations or the like by prices, you rank job applicants by their qualifications; and “the marriage problem,” where you rank suitors by eligibility, for two. All incarnations share the same underlying mathematical structure, in which a known number of rankable opportunities present themselves one at a time. You must commit yourself to accept or reject each of them on the spot with no take-backs (if you decline all of them, you’ll be stuck with the last choice). The opportunities can arrive in any order, so you have no reason to suspect that better candidates are more likely to reside at the front or back of the line.







/cdn.vox-cdn.com/uploads/chorus_asset/file/25831586/STKB310_REDNOTE_XIAOHONGSHU_B.jpg)